

Types of operations are expressed as + (addition), - (subtraction), *(multiplication), / (division).There are a few things to note when defining functions: You may want several input values, and you may want the user to group some of those input values in curly brackets. Instead, you may need to carry out several steps of computation, using temporary variables. However, in may cases, you may find it impossible to define the function's value in a single simple formula. The simplest user-defined functions are the "one-liners", where the quantity of interest can be computed by a single formula. \) in Mathematica the correct syntax is f. That is, while in mathematical notation, we write \( f(x), This is like how we suppress the result of a command by typing = as was described earlier.īrackets. To suppress output, type a semi-colon ( ) at the end of input of your command. However, if f=3*x+1 is entered, then f is 7, f = 3a+3b+1, and f is 3x+1 because entering x_ allows me to plug in different values for "x-blank." To indicate that the argument is the product ofĪ and t. Ordinary parentheses are used exclusively for algebraic grouping. That is, while in mathematical notation, we write \( f(x), \) The symbol x_, pronounced ``x-blank,'' denotes a ``pattern'' named "x." For example, If one were to enterį =3*x+1, then f and f will not be evaluated, but g will be evaluated because x is set as the only variable that this function will accept.įunction evaluation in Mathematica is indicated by squareīrackets. Return to the main page for the course APMA0340 Return to the main page for the course APMA0330 Return to Mathematica tutorial for the second course APMA0340 Return to Mathematica tutorial for the first course APMA0330 Return to computing page for the second course APMA0340 Return to computing page for the first course APMA0330 Laplace transform of discontinuous functions.Picard iterations for the second order ODEs.
#FUNCTION MATHEMATICA SERIES#
Series solutions for the second order equations.Part IV: Second and Higher Order Differential Equations.Numerical solution using DSolve and NDSolve.Part III: Numerical Methods and Applications.Equations reducible to the separable equations.The Primary Course by Vladimir Dobrushkin, CRC Press, 2015 This tutorial is accredited appropriately. The right to distribute this tutorial and refer to this tutorial as long as
#FUNCTION MATHEMATICA FREE#
You, as the user, are free to use the scripts for your needs to learn the Mathematica program, and have
#FUNCTION MATHEMATICA LICENSE#
While Mathematica output is in normal font.įinally, you can copy and paste all commands into your Mathematica notebook, change the parameters, and run them because the tutorial is under the terms of the GNU General Public License
The Mathematica commands in this tutorial are all written in bold black font, It is primarily for students who have very little experience or have never used Mathematica and programming before and would like to learn more of the basics for this computer algebra system.Īs a friendly reminder, don't forget to clear variables in use and/or the kernel. Posted in Economics, International Trade, Mathematica and tagged Mathematica, piecewise functions, plot, relative supply, Ricardian model on Octoby Paz, Lourenco S.This tutorial was made solely for the purpose of education and it was designed for students taking Applied Math 0330. You can find more information about these options at. Note also that in the example above I utilized several options of the command Plot. Plot is for a function that takes the value 15 if x is smaller than or equal to 5 and the value 3x if x is greater than 5.
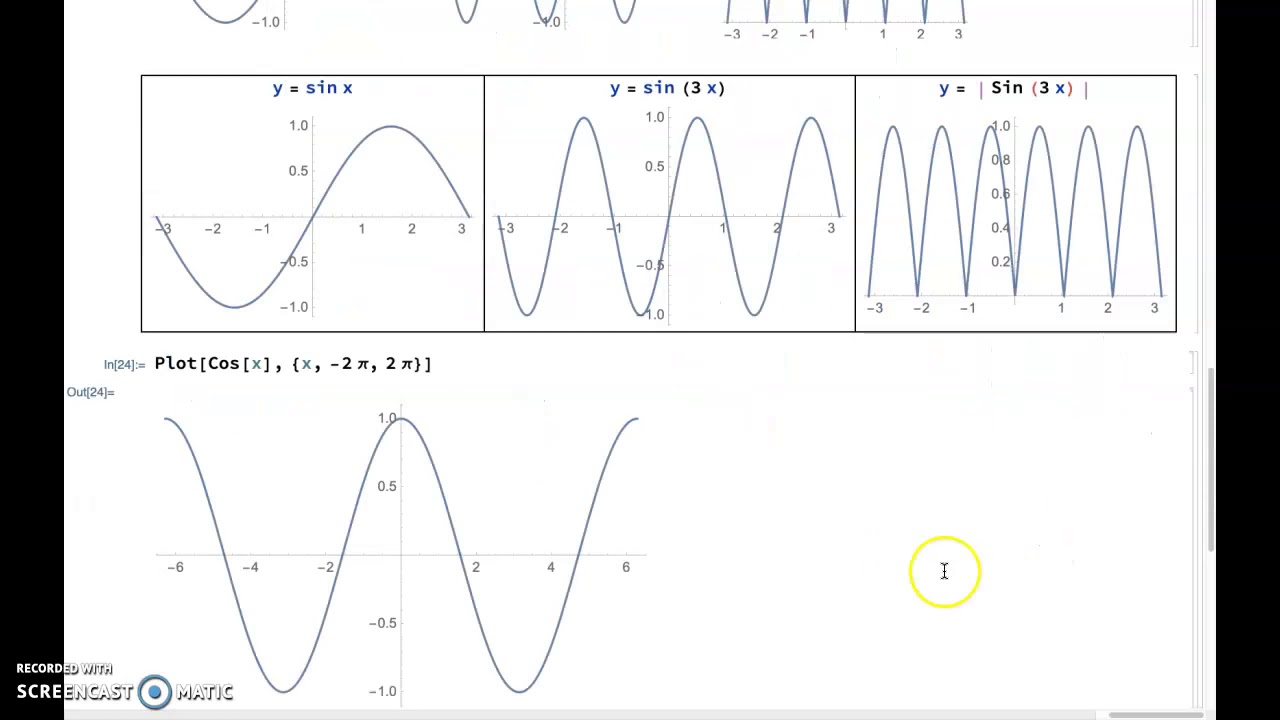
Below you can find an example to illustrate this: Those interested in representing graphically the Relative Supply (RS) curve of a simple 2 x 2 Ricardian model of international trade in Mathematica will find that this task requires the use of Plot and Piecewise.


 0 kommentar(er)
0 kommentar(er)
